In a video by Joe Scott I recently ran into the following statement:
Magenta should be a combination of red and blue, but the half way point between red and blue on the spectrum is green. But obviously we don’t see green, we see a color that is nowhere on the light spectrum. Our brain just makes it up. So even though magenta is one of the primary pigment colors that you can combine with the other colors to make all the different colors that we experience out there in the world, it doesn’t have an actual wavelength associated to it. It is completely made up in our brain.
What If You Could See Every Wavelength Of The Electromagnetic Spectrum? | Answers With Joe – Joe Scott – 9 Sept 2019 – https://youtu.be/Dq9SU5dUj48?t=126
A while later I heard the following statement:
Magenta is not actually a color.
Author omitted.
This did not sit well with me, but as it was a verbal conversation I did not have the time nor resources to figure out exactly why magenta should be considered a color, despite not having it’s own wavelength. Overthinking this on a sleepless night led me to the following hypothesis:
Hypothesis
Magenta does exist. Color should not be seen as an average of frequencies, but as a signal. The individual color receptors in our eyes then filter out given frequencies, but are also triggered by frequencies close by. As such, a composition of two adjacent frequencies (red and green or green and blue) is, to our eyes, indistinguishable from the average of those frequencies. However, a composition of two frequencies that are not adjacent (red and blue) is distinguishable from the average of those two frequencies.
This would consequently mean there are two types of yellow, which our eyes can not distinguish. One type would be a single wavelength between 590 and 560 nm, the other type would be a combination of red (700-635nm) and green (560-520nm). These two types of yellow would be indistinguishable from each other, as they both trigger the red and green receptor in our eyes by an equal amount. Magenta is however distinguishable from green, as although it triggers the red and blue receptor an equal amount, the lack of trigger of the green receptor causes our brain to differentiate between green and magenta.
This also implies that tetrochromic people can distinguish between the aforementioned two types of yellow, as the fourth receptor will be triggered a different amount. This phenomena has been reported, as tetrochromats report colours not matching while to a trochromat they do match. (Source: “The women with superhuman vision – BBC Future” https://www.bbc.com/future/article/20140905-the-women-with-super-human-vision)
How cones work
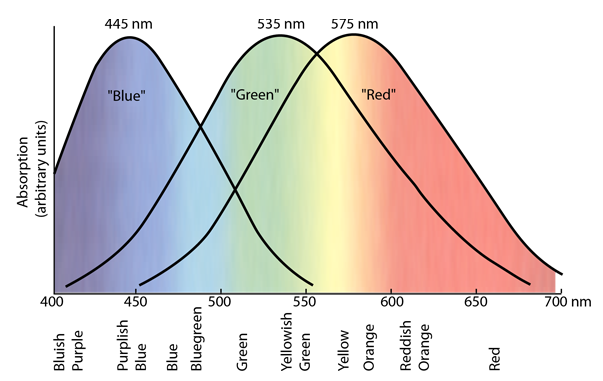
To confirm this hypothesis, we first need to understand exactly how cones work. My initial assumption here is that cones get triggered when a given frequency is present in a combined signal of frequencies. A quick search here turns up the following image:
What is important to note here is that although the peaks are drawn at equal heights, this height is arbitrary. Blue cones only make up about 2% of the cones, but seem to somehow be amplified. As such, it’s not easily possible to map a given amount of light in lux at a specific frequency to a given response in the receptors in the eye, and the numbers will remain arbitrary. For our current purpose, we will assume that a given amount of light at the blue frequency will trigger a response of the same intensity as the same amount of light in the green frequency.
Something else that is interesting to note, is the names of the colors right below the highest point of the peak. I would personally have assumed that when the red receptor (575nm) is triggered the most, you’ll see red. This seems to not be the case, as the word red is positioned around 660nm, and the frequency 575nm actually shows as yellow. (Or well, 580nm shows as yellow, but close enough.) This is counter intuitive to me, as I had the following image in mind:
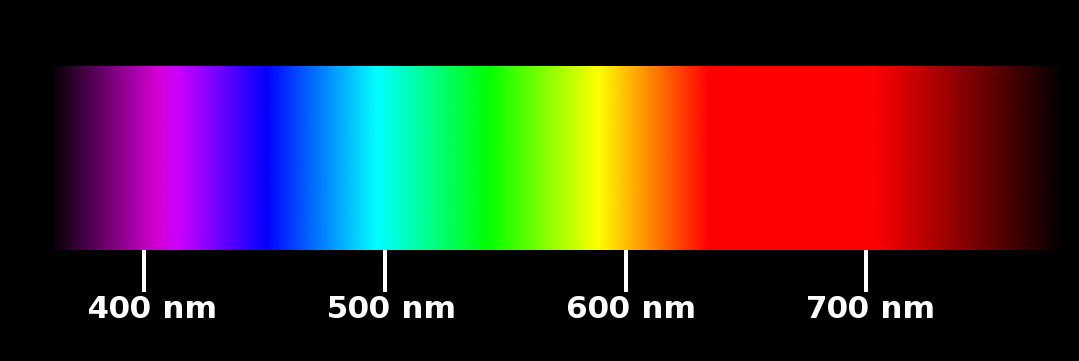
My personal assumption was that the red receptor would detect 700nm, green would detect 550nm and blue would detect 450nm. I was wrong there. Instead, red seems to be detected by “a presence of something near the red receptor (575nm), but far from the green receptor (535nm).
How does mixing colors work
So to recap, when light of a given frequency hits a cone, it will be triggered. The cone is not only triggered by that specific frequency, but the closer it is, the “more triggered” it gets. But if the “blue” receptor detects 445nm, and the green receptor detects 535nm, how are the colors in between detected?
■ 445nm light, aka violet
■ 535nm light, aka green
As you can see above, blue is not directly detected. The color blue has a frequency of 465nm.
■ 465nm light, aka blue
This means that, to perceive blue, multiple receptors will get triggered. I do not have exact numbers and will be making measurements from the image above. When blue light enters the eye, the green receptor gets triggered at roughly 30% “capacity”, and the violet receptor gets triggered at roughly 95% capacity. This is what our brain interprets as being “blue”.
Now let’s see how green is detected. Green seems to trigger all 3 receptors, so let’s first establish what the third receptor is.
■ 575nm light, aka yellow
As stated before, green has a frequency of 535nm. This seems to be the only receptor where the color actually of the receptor actually matches the primary color. Measuring from the image above, with 535nm light, the violet receptor gets triggered at around 15%, the green receptor gets triggered at 100% (obviously), and the yellow receptor gets triggered at 62%.
Is red a color?
In the previous paragraph, we stated that the three receptors in the eye actually capture violet, blue and yellow light. What is striking to me is that the cones only cover the given area of the spectrum, as outlined with a white square:
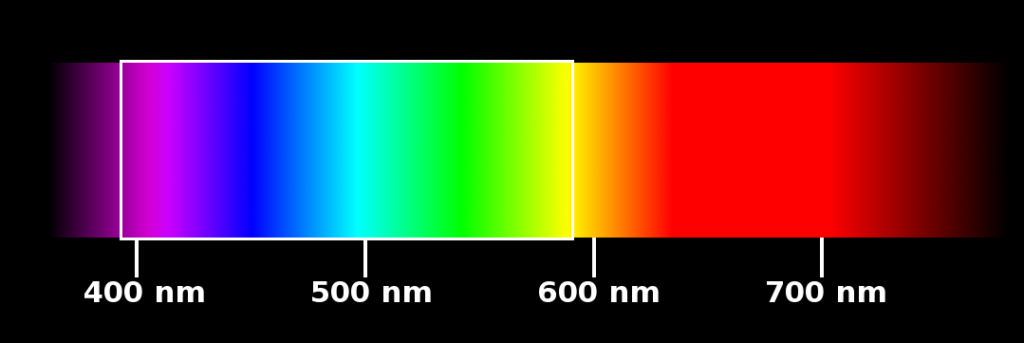
Red is not a color we can actually perceive directly. There is no cone that gets triggered by something being “red”. But then how come that we do see red? Let’s look at image 1 again. According to wolfram alpha, red starts at 620nm, and tapers off outside of the visible spectrum. So let’s assume a frequency of 620 for red. Measuring from image 1 this gives us a response on the yellow cone of 57%, and on the green cone of 22%. Our brain then interprets this ratio of green to yellow as “red”. So in other words, detecting something on the yellow cone while not detecting as much on the green cone means something must be red.
Red is not actually detected by any cone in our eyes! It’s the combination of the green and the yellow cone in a specific ratio that makes us believe we see red!
Fake red?
I will continue diving into this some other time.